Capacitor with Dielectric
What is a dielectric
A dielectric material is an insulator in which there are no free electrons.
This means that a dielectric will not be able to conduct current.
Dielectric constant
Dielectric constant K can be written as a ratio of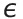 
Permittivity of the medium
to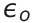 
Permittivity of free space.
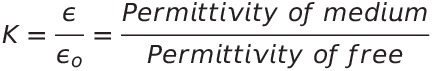
It can also be defined as the ratio of 
the
capacitance with dielectric as the medium and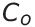 
is the capacitance with air or vacuum between the plates.
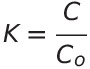
Further it can also be defined as the ratio of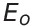 
the
electric field between the plates with air or vacuum as the medium and 
is the electric field between the plates with dielectric as the medium.
Polar and non-polar materials
Materials can be categeorized into polar or non-polar materials.
Polar materials
Materials and called polar materials when the molecules have a net dipole moment. This means that the center of mass of the positive charge and the center of mass of the negative charge in the molecule are slightly seperated
Non-polar materials
Materials and called non-polar materials when the molecules do not have a net diapole moment. This means that the center of mass of the positive charge and the center of mass of the negative charge in the molecule concide and are not able to create a dipole moment
Atoms due to their spehrical symmetry are always non-polar
Capacitor with dielectric
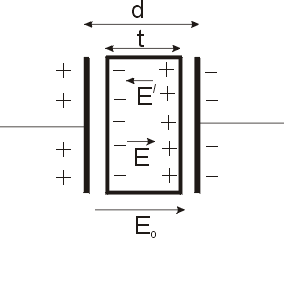
If we place a dielectric between the parellel plates of a capacitor and give a charge
to the capacitor then in the absence of a dielectric there is an electric field
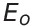. Say the distance between the plates is d
and the thickness of the dielectric is t. Now, since positive charge is on one plate
and negative charge is on the other plate, it is going to induce diapole moment in the molecules
of the dielectric. If the molecules were initially non-polar they will have this induced however, if
they already had a diapole moment they will realign according to the positive and negative charge
on the plates, thus orienting themselves in the direction of the electric field. Now the left
side of the dielectric will have a net negative charge and ther right side a net positive charge. And,
as a result the direction of the induced electric field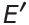 
inside the dielctric will be from right to left.
So since there is an electric field to the left due to induction, the net electric field
inside the dielectric will reduce and it will be:
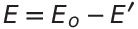
However the direction of
will be the same as the direction of
Effect of a dielectric on the capacitance of a capacitor
Let 
be the area of each plate
In the absence of a dielectric the capacitance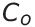
can be written as:
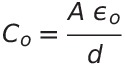
Once the dielectric having a thickness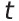 
is placed between the plates the capacitance will change.
the potential between the plates will be:
Here in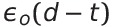 
is the product
of electric field 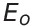and the distance
of space free of the dielectric, which is given by
.
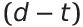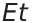is the product of the electric field
inside the dielectic and the thickness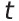
of the dielectric.
Now
therefore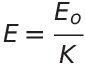 
we know that
therefore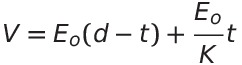 
or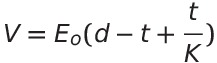 
From Gauss's Law we know that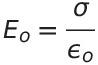 
where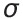 
is a the surface charge density, that is
the charge per unit area
so we can say that if 
is the charge
on each plate, that is negative on one and positive on the other, then
or 
we know that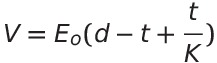
therefore 
hence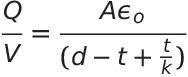 
we know that capacitance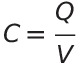 
hence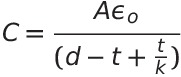
This is the formula of capacitance with a dielectric which is not completely filling the plates
From here we can see that if the dielectric completely fills the plates then t will equal d. When we subsitute this then we get
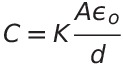
we have seen that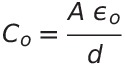
therefore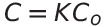 
Now as K is always greater than 1 that is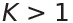 
it emplies that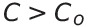 
Or if we put a dielectric between the plates of a capacitor then its capacitance is going to increase
Dielectric strength
It is the maximum value of electric field that a dielectric will be able to tolerate without electric breakdown.